
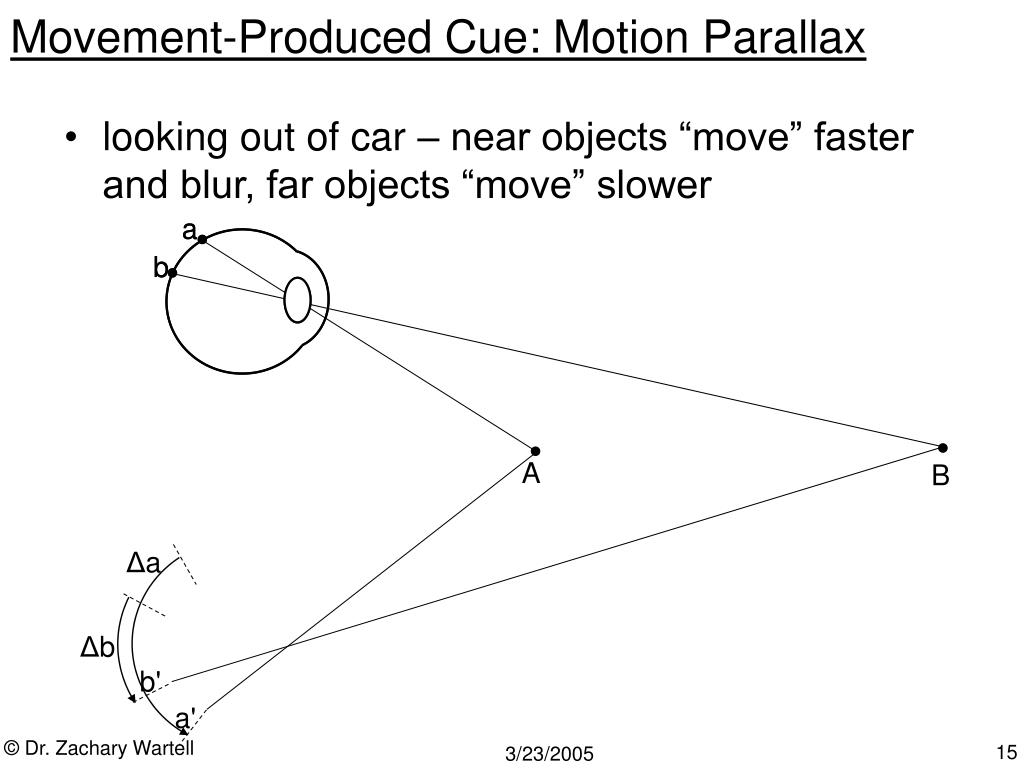
However, most stars even in our own galaxy are much further away than 1000 parsecs, since the Milky Way is about 30,000 parsecs across. Space based telescopes can get accuracy to 0.001, which has increased the number of stars whose distance could be measured with this method. This limits Earth based telescopes to measuring the distances to stars about 1/0.01 or 100 parsecs away. Parallax angles of less than 0.01 arcsec are very difficult to measure from Earth because of the effects of the Earth's atmosphere. Limitations of Distance Measurement Using Stellar Parallax This simple relationship is why many astronomers prefer to measure distances in parsecs. The distance d is measured in parsecs and the parallax angle p is measured in arcseconds. There is a simple relationship between a star's distance and its parallax angle: d = 1/ p Stellar parallax diagram, showing how the 'nearby' star appears to move against the distant 'fixed' stars when Earth is at different positions in its orbit around the Sun. The star's apparent motion is called stellar parallax. Astronomers can measure a star's position once, and then again 6 months later and calculate the apparent change in position. As the Earth orbits the Sun, a nearby star will appear to move against the more distant background stars. This effect can be used to measure the distances to nearby stars. Compare the results of (a) and (b) to those of the earlier exercise on the said page number. Find the p-value in (a) and interpret its meaning. Your hand will appear to move against the background. a depth cue whereby a difference in the apparent rate of movement of different objects provides information about the relative distance of those objects. We would then adjust the pin by moving it forwards or backwards until the object and image moved at the same speed, just like in the video below.Another way to see how this effect works is to hold your hand out in front of you and look at it with your left eye closed, then your right eye closed. All we have to do then is put ourselves behind the pin so that we can view both the real pin and the image of the pin (as reflected through the mirror) and move our head left-right to see how the object and image move relative to one another. they appear to be moving at the same speed as each other) then they must be in the same location. We know that if an object and an image have “no parallax” (i.e. This is because with positiviely powered mirrors, when an object is placed at the centre of curvature, the image forms in the same location, but inverted.īut how do we determine where the location of the image in the mirror is? Well, this is where the “no parallax” test will prove to be useful. Now in order to locate the centre of curvature and therefore, be able to measure the distance of the radius of curvature, we need to get the object (the real pin) and the image of the pin to be in the same location. a pin) facing the reflective side of the mirror, as demonstrated below. To utilise this principle to help us locate the centre of curvature of a concave spherical mirror, we need to place an object (e.g. No parallax is only evident when both objects are in the same location.No parallax is only evident when both objects are moving at the same speed.This reveals two important features of the “no parallax” test: The way to get your fingers to have “no parallax” is to put them side-by-side so that they move at the same speed as each other. This is because your fingers are in different positions relative to your head and therefore they have parallax.
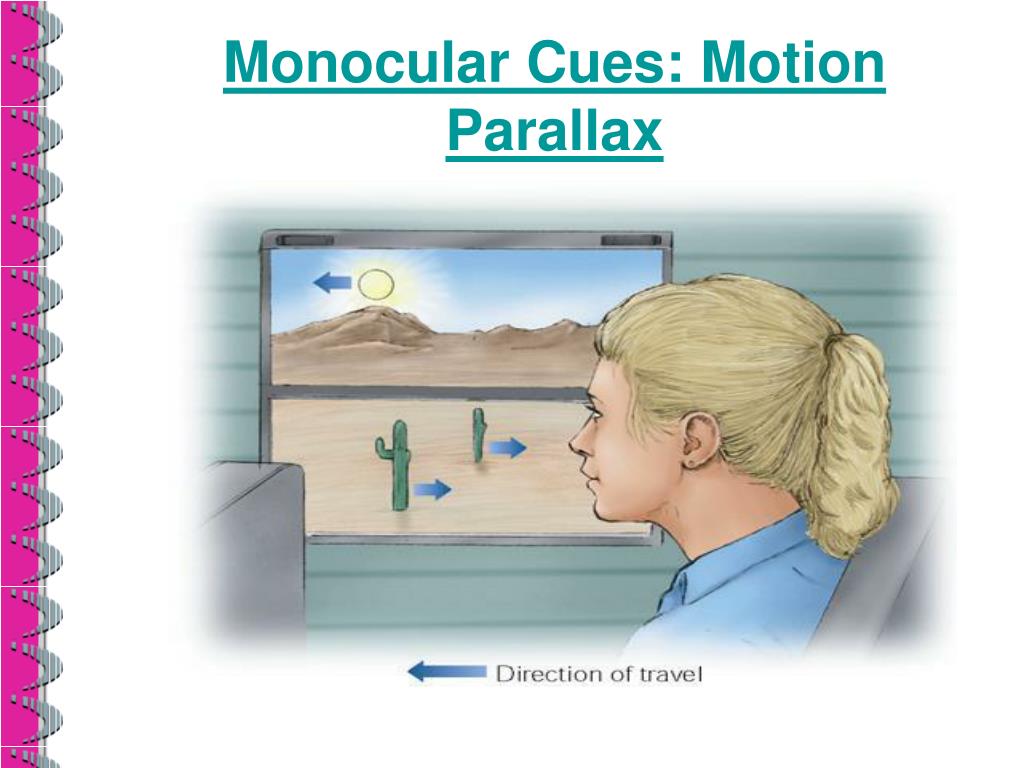
Hopefully you’ll observe that the finger closest to you appears to “run past” the finger that’s further away – as you move your head left, the close finger will “run” past the distant finger to the right, and the opposite will happen when you move your head to the right. Now move your head slowly left and right whilst keeping your fingers stationary (this is easiest to do if you focus on the finger that’s far away). Firstly, hold the index finger of your left hand near your face and hold the index finger of your right hand as far away as you can reach. To illustrate this, I need you to do a little demo at home for me. Parallax is the term used to describe the error in position of objects due to their relative position to us.


 0 kommentar(er)
0 kommentar(er)
